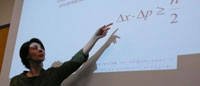::: center home >> being here >> last donut? >> structural |
16 January 2009 Pittsburgh has long been steeped in scientific explanation. When I came here in the early 1980s, on the faculty were both Carl ("Peter") Hempel and Wes Salmon, the founders of two major traditions of thought in scientific explanation. Today I was pleased to see the tradition continue in the work of one of our Visiting Fellows, Laura Felline from the University of Rome.
Laura began by mapping out the curious situation of explanation in quantum theory. Three quarters of a century after the theory found a roughly stable form, we still do not know precisely what the theory says is in the world. This embarrassment is dignified in philosophy of quantum theory by calling it the problem of "interpretation." The theory talks of photons of light as particles. That calls to mind the little corpuscles that were so popular in seventeenth century natural philosophy. But light, it says, is also extended in waves, with frequencies and wavelengths. Somehow both have to be true at the same time. Whatever that may mean, both cannot be true literally. It is not just light that has this odd, dual character. It is attributed to all bodies treated by quantum theory: electrons, protons, atoms, molecules, specks of dust, elephants and planets. So, Laura tells us, philosophers allow that quantum theory makes predictions of extraordinary accuracy. Yet they regard the theory as explanatorily mute. How can the theory explain when we do not know what the theory says is really there? The attitude of the physicist is quite different. They are unashamed in their belief that quantum theory can explain lots and beautifully so. "Why is there this chasm?" she asks us.
Why the chasm? Her answer is simple. The physicists got it right; the philosophers got it wrong; and she is going to explain how.
I picked up at Rob's name. Rob had been our colleague here in Pittsburgh for a few short years. He had a razor sharp philosophical mind and a talent for engaging and enthusing students that I have never seen equaled. Illness took him at 39 years of age, but we have never forgotten him. It was good to hear from Laura that his clear voice is still heard beyond our halls. The basic idea of structural explanation is simple. Merely displaying mathematical relations within a theory, it says, is good enough for us to say that quantum theory explains. She did have a more precise recipe for just how these relations are to be displayed and how they relate to the explanandum, the thing to be explained. You can read those details in her papers. What gives a clear sense of how it works is the example she proceeded to display. Famously, in 1927, Heisenberg noted that the new quantum theory entailed a reciprocal uncertainty in the position and the momentum of a particle. The more precisely specified the position, the less precisely specified the momentum; and conversely. All this is neatly wrapped up in a familiar formula with deltas and x's and p's and h's. Heisenberg explained this reciprocal uncertainty by means of a microscope. If we wish to know where a miniscule body is, we must shine a light on it. The light consists of a stream of photons that will bounce off the body. By collecting the scattered photons in a microscope, we can determine the body's position. Now comes the complication. Photons carry momentum, so their impact will alter the momentum of the particle. We can minimize this disturbing effect by using long wavelength photons, which carry less momentum. But the trouble with using long wavelength photons is that they are more spread out in space. Our microscope's discrimination of the body's position will suffer correspondingly; it is just nineteenth century optics. We see the reciprocity in this trade-off. If we use short wavelength light to illuminate the body, we can locate its position precisely, but we will uncontrollably alter its momentum. The more we seek to leave the momentum unaffected by using longer wavelength light, the less precisely we learn the position. The story of Heisenberg's microscope is simple and appealing and has appeared in many text books. The uncertainty relations are explained by the familiar repertoire of the nineteenth century physics: little corpuscles in motion, waves, their scattering from a body and the resolving power of microscopes. The trouble is that is just complete bunk. Or at least that is what most accounts of the foundations of quantum theory will tell you. Heisenberg's thought experiment suggests that the little body has both a definite position and momentum. It is just the ham-fistedness of our instruments that preclude us learning both precisely. Most accounts of the foundations of quantum theory tell us that the body just doesn't have both a well-defined position and a well-defined momentum. At least one of them is a quantum blurr with no definite value. Nonetheless Heisenberg had found a relation that is central to quantum theory. There is a reciprocal relationship between the quantum blurring of position and the quantum blurring of momentum. We end up in misleading fictions, Laura is tell us, if we try to use the nineteenth century explanatory instruments of Heisenberg's microscope to understand it. Moreover, our understanding does not require these instruments. There is a structural explanation that does the job of explaining perfectly well. We see the quantum blurr in the position of a particle when we represent its wave function as spread out in position space; we see the corresponding blurr in momentum when we represent its wave function as spread out momentum space. What carries us between the two descriptions is a familiar piece of mathematics, the Fourier transform. The reciprocity of Heisenberg's relations results directly from the mathematical properties of this transform. A particle with a definite position is represented by a spike in position space. Its Fourier transform is a widely spread wave in momentum space. And the reverse holds as well.
There was much more to say. She delved into just what mathematics she had in mind and then into another example concerning the explanation of length contraction in special relativity. Some 35-40 minutes into the talk, she finished.
We were reseated for questions. Before the first question was asked, I had a list of seven names on my notebook. The questions were varied, but as they unfolded, I began to see a skeptical gap opening between Laura and the room. Peter Machamer, perhaps, put the worry most succinctly, at the end of a longer speech. Did Laura really think that merely displaying mathematical abstractions is providing understanding? I was listening as Peter spoke and noted that he artfully avoided his favorite word "mechanism," although I do think that "causal" crept in. What could he mean? If mathematical abstractions could not explain, what could? Well, I was sure I knew what Peter was thinking. You explain by displaying the causal mechanism. Laura later told me this was a great moment. She had been nervous presenting the talk. But now it all evaporated in the face of a definite challenge before her. She brought to mind the seventeenth century account of inertial motion. Prior to Newton and Galileo, one needed a cause to explain why something might persist in moving uniformly in a straight line. After that, it was a primitive mathematical fact of the new physics. We accepted, eventually, that there was nothing more to understand about it. No cause need be given and none could. This was a moment for me too. It somehow crystallized thoughts that had been lingering in my head. The mistake in the room was to think of explanation as a single, pure and perfect notion that lives outside any particular theory and is to be realized in each, in pretty much the same way. No, each new theory brings its own mode of understanding, and with its own notion of explanation. They may not differ much from theory to theory. Or they may. In one science, displaying causes and mechanisms might be the way. In those parts of quantum theory considered by Laura, understanding comes in a different mathematically grounded form and that fact must be reflected in any account of explanation applicable to that domain. When finally my turn came, that was my speech to the room. When our immovable stopping time of 1:30 came, I presented Laura her umbrella and explained how the telescopic drip guard works. That familiar ritual did not stop the discussion. Laura was trapped at the lectern by questioners. The herd thinned, but, by the time I left, she was still surrounded by virtually all the visiting fellows of this term, eagerly continuing the discussion. John D. Norton Lunchtime Talk |