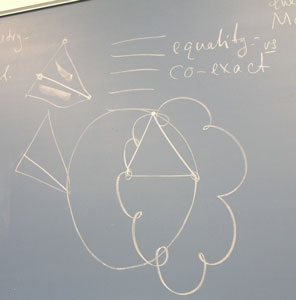![]()
::: postdoc fellowships
::: senior fellowships
::: resident fellowships
::: associateships
![]()
being here
::: visiting
::: the last donut
::: photo album
::: center home >> being here >> last donut? >> 27 October 2006 |
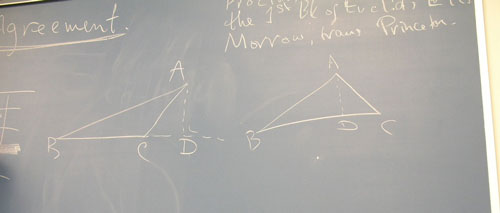
Friday, 27 October 2006 Ken Manders, our speaker today, has been thinking deeply about geometric demonstrations and the role of figures in them for years. He has come a few minutes early to our seminar room to set up. That meant laying out a few texts for easy reference. I notice Euclid's Elements, the ancient treatise of thirteen books that defined geometry for milennia. What audiovisual aids does he need, Joyce asked, looking up from her artful arranging of the donuts and bagels. None? Hmm. How refreshing! Quite a change, I quipped, from what so many do. Elaborate productions. Contracts with Spielberg. A year's lead time. Ken smiled politely and turned his head into his book. The talk began with Ken standing before a blank chalkboard. His topic was Euclidean geometry--more specifically the geometry of the first few books of Euclid's Elements. He reminded us of a talk he'd given 10 or 15 years before here on the same topic. His concern then was to understand just how Euclid's geometrical demonstrations worked, urging that we had lost something in moving away from them. As Ken spoke, he paced up and down, turning to the chalkboard to write key words or sketch the geometric figure at issue. That was bucking the trend of audiovisual aids. And I didn't mind a bit. The presentation was every bit as interesting and dynamic as the slickest Powerpoint. Ken was also bucking a second, more serious trend. The dominant tradition in modern proof theory regards Euclid's efforts as inspired but ultimately flawed. For they depended essentially on the diagrams that accompanied the text. The worry was that the proof required some sort of ineffable geometric intuition. What would happen if you or I see the same figure but just have different intuitions? Why wouldn't the proof dissolve? That is an unacceptable fragility. Modern proof methods in geometry had completely done away the diagrams and thereby proclaimed the problem solved. Ken recalled why this view is mistaken. Over roughly two thousand years, during their transmission from culture to culture, Euclid's proofs had never engendered the confusions modern theorists feared. This was from ten years ago. The new material today turned on the notion of objections to steps in the proofs. Ken began to recount an ancient tradition of objections. Once again the board filled with figures of triangles and circles. Ken was doing things that couldn't easily be done with Powerpoint. A line needed to be broken. He deftly rubbed out bits with his finger. The whole line needed to be replaced. It was gone in a second with a finger wipe and a new one drawn in. I can do that in Powerpoint too, but I first need to draw figures in a drawing program and then implore Powerpoint to deal with them just as I want. Ken had demonstrated the entire effect before I'd even figured out how I'd do it in Powerpoint. The objections were of a familiar type. If the proof requires you to drop a perpendicular to the base of a triangle, you might be wrong to assume that the point so defined lies on an extension of the base. Or if you draw a chord to a circle, how do you know the chord lies within the circle? Those assumptions are routinely made in a Euclidean proof. Each can be challenged. But why is Ken now talking about the ancient tradition of objections? Didn't he have enough in dealing with the modern objections? As the examples multiply, his point gradually settles in. This tradition of objection is actually a part of the Euclidean proof tradition that we'd been overlooking. Euclidean proofs have a dynamic character. Unlike the perfected proofs of the modern literature, Euclidean proofs live and evolve. As these ideas tumble into the room, the chalkboard fills with figures; they are erased; and the space is filled again. I watch as Ken's fingers become covered in chalkdust and his pants attract more chalky smudges. He has brought a cup of tea, which he picks up to sip, but the thoughts are coming so constantly that he cannot pause and the tea is carried back and forth across the room before the sip finally is taken. So, if Euclidean proofs live and evolve, why should we ever feel comfortable with one? Why can the geometers ever rest? Now came the final piece. As long as the geometric problems are of the simple type Euclid addressed, only finitely many objections are possible. The point of intersection may lie within the triangle or outside it or on the edge. That's it. Just three possible cases. So, for any proof, there can only be finitely many objections. The process of objection and reply or repair cannot continue indefinitely. While we may not know it, the process may already have come to an end. To someone like me, who is steeped in the logical tradition of proofs as fixed series of symbols, this new view of Euclid's methods as living and dynamic is quite a revelation. It should not have been, Ken hastened to point out. For that is how real mathematics is done today. All modern proofs start out as sketches that are run past friends, who object and demand repairs; and then past referees, who may do the same. Indeed we all recall how the proof of Fermat's last theorem was announced but then flaws were found and we waited anxiously for months to hear if the proof could be repaired. Ken's story came to a close, reminding us that much needs to be done to get a more precise image of just how this cycle of objection and repair proceeds in Euclid's geometry. But his time was up and there was no doubt that he had earned his umbrella. John D. Norton Kenneth Manders |