::: center home >> being here >> last donut? >> similarity and dimensions |
Dynamically Complex Behavior
Friday, 22 January 2010 Our talk today in the lunchtime series comes from a new Fellow, Meinard Kuhlmann. The project he'd foreshadowed for this term was in foundations of quantum mechanics. So the subject of today's talk, complex behaviors, was unexpected. It should not have been so unexpected. Each year we assemble an energetic community of scholars in the Center. They rarely have just one interest and by the time they are ready to stand in front of us and speak, their enthusiasms have moved along. This keeps things exciting for me--I never quite know what they will do next. Meinard's topic proved to be especially timely. In less that two weeks, we would host a small workshop on Sandy Mitchell's Unsimple Truths, which is a philosopher's view of complexity. Today's talk would be a warm up for it. There was more as I found when I chatted with Meinard ahead of his talk. He planned to relate complex behaviors to mechanisms. The 2000 paper describing the Machamer-Darden-Craver ("MDC") account of mechanism was still topping the list of the most cited papers in the journal Philosophy of Science. We'd heard a great deal about MDC at the "Peterfest" held in honor of Peter Machamer in the same room just a few months ago.
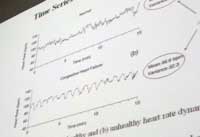
Philosophers love to divide and categorize. That was Meinard's starting point. There are, he urged, two sorts of complexity. One is "compositional" and is found when one has a system consisting of very many components. The complex behavior arises from their interactions and in a such way that small changes in one component will have a big effect. This sort of complexity is visible in space in the complicated layout of the components. Another sort of complexity is visible in time. One can have quite simple systems whose dynamics is complex. Meinard made the point by displaying a compound pendulum. It has a few very simple components, but a complicated behavior in time. As anyone knows who has watched them in action, they seem to jerk and toss about as if animated by a hidden will. This was interesting. While I'd always known of the multiple senses, I'd never thought explicitly about the difference. But two remarks stopped me. Meinard characterized biologists as working only with compositional complexity. That was a natural assumption since compositional complexity is exactly what makes understanding organisms hard. But I wondered if they so fully ignored dynamical complexity. Sandy is an expert in both complexity and philosophy of biology and I could see her shifting in her seat. My thoughts jumped forward to question time. The other remark was that dynamically complex systems are insensitive to small changes in their parts. That didn't fit with what I knew of chaotic systems. They were defined as those whose behavior exhibits sensitive dependencies. I made a mental note to see how Meinard would handle this. Meinard proceeded with two examples, passing to the property they displayed that especially interested him. This was the notion of self-similarity. It arises in a time development when the statistic for a long period of time look the same as for a short period. That is,informally speaking, you cannot tell if the history just given to you is for a week or a day. This kind of self-similarity arises in the first example of heart beats. A graphical plot of a healthy heart beat is self-similar in time. It has no characteristic time scale. Instead, it has that typical fractal look of curves arising in complex systems. However the heartbeats of people with congestive heart failure were not fractal--they do exhibit a definite periodic form with its own time scale. The second example was prices on the stock market. They too fluctuate in time, following fractal self-similar statistics. The important point in this example is that the size of fluctuations of such systems are much greater than one would routinely expect. They diminish in probability according to a power law in their magnitude, whereas we naturally presume a rapid exponential decline of the familiar Gaussian normal curve. That power law, Meinard explained, meant that wild fluctuations--stock market crashes--are much more likely than we think. Given the turmoil in the world economy of the last year or so, it was a timely example. But it gave me no assurance that econophysics, from which the example was drawn, was so prescient. If those fluctuations were to be expected, why did all the Wall Street geniuses and their econophysicist advisors seem so surprised? I now understood Meinard's earlier remark about insensitivity of the complexity to small changes. While the precise price of some particular stock may alter if we make a tiny change in the background conditions, that change will not alter the power law statistics. That is the insensitivity he intended. We were now well into the talk and I had been enthralled by the illustrations of complexity. But when would the philosophy start? It now began. When the analysts find this fractal behavior in heartbeats or market prices, they are happy to exclaim that they have found the mechanism that explains the behavior. That, Meinard the philosopher observed, was far too quick. Observing a power law statistics or scale freedom is interesting and may be the beginning of something, but it is not explaining. How might we go about explaining these features? We should find a mechanistic explanation, for that is what the scientists themselves claim that they have found. But what sort of mechanism? Meinard was now proceeding cautiously. It would not be the mechanisms of MDC he conceded. Knowing Peter Machamer sat within heckling distance, he moved on quickly. Meinard sought a more structural notion of explanation. The illustration of this structural approach was the use of symmetries in quantum theory. Meinard's brief excursion into structural explanations in quantum theory was comfortable and familiar. It had been almost exactly a year ago that Laura Felline, a Fellow from last year, had stood in the same spot and explained how structural explanations in quantum mechanics work. Meinard, I later found out, knew of Laura's work here and, as a result, presumed mistakenly that he needn't explain the notion too deeply. It had been a rich and interesting talk. The time had passed quickly and now it was over. Yet somehow it was over too soon. In the few minutes break before question time started, I wandered up to Meinard. "Very nice talk! But I still don't know what you mean by structural mechanism," I remarked. Meinard didn't hesitate in replying. "I didn't say. Figuring that out is the project here. Perhaps I should have made that clearer!" We began to pursue these thoughts when Balazs wandered past and complained that I was asking questions in private--no one else gets to hear them. I was pretty sure now that the incomplete specification of this structural notion of mechanism would figure prominently in the question time. It did. First Sandy corrected Meinard on his narrow portrait of the biologists' conception of complexity. Then, she pressed him to say what assumptions are needed to get deeper understanding from the statistics. Meinard had now taken a seat at the front of the room and jotted notes as she spoke. Now it was Peter Machamer's turn. Why, he wanted to know, wasn't the MDC model fully up to the task. As he and Peter exchanged their remarks, it was clear that there was a distance between them that was not closing. That gap depended, it seemed to me on a different readings of the term "mechanism." To Peter, the term was being used for a fundamental unit of scientific theorizing, such as a basic mechanism of cell biochemistry. Meinard's use was more metaphorical. It referred to characteristics of a computation and why it might produce the results it does. How is it that the statistical averaging of the behavior of many traders produces a power law curve? There is some property of the system that, when fed into the computation, assures a power law. The affect of that property on the computation is, to speak as I guessed the scientists might, the "mechanism" that produces the power law. I abused the chair's privilege to interject and try to close the gap.
When it was all over I stood in the emptying room with Meinard, assuring him that he has a great project and that I look forward to thinking about what general features a system must have to manifest the dynamical properties at issue. We had scheduled our next Fellows' reading group on his work, with the expectation that we would have plenty to talk about after hearing him speak. It was now clear that expectation was correct. John D. Norton ::: Lunchtime Talks |