![]()
home
::: about
::: news
::: links
::: giving
::: contact
![]()
events
::: calendar
::: lunchtime
::: annual
lecture series
::: conferences
![]()
people
::: visiting fellows
::: postdoc fellows
::: resident fellows
::: associates
![]()
joining
::: visiting fellowships
::: postdoc fellowships
::: senior fellowships
::: resident fellowships
::: associateships
![]()
being here
::: visiting
::: the last donut
::: photo album
|
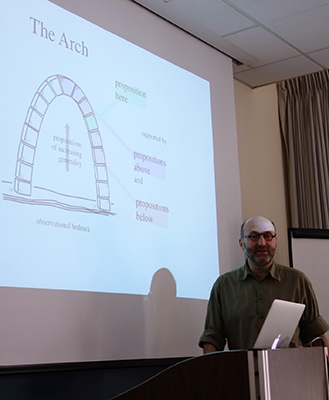
Induction Whether a talk is successful or not depends on a great deal more than the content of the talk and the skill of the presenter. A talk is, in the end, an interaction between the speaker and the audience. As with any human interaction, whether it succeeds depends on both sides. That was the thought as I headed in to give my talk today here in the Center. I suspected that I was heading for trouble, double trouble. Let me list them. First, among those who actually think about inductive inference, there is a newly formed orthodoxy: inductive inference just is probabilistic inference. There are few problems, according to this view, that an application of Bayes' theorem won't solve. And a double dose, a second application, will deal with the few recalcitrant remainders. Problems of inductive inference are now no worse than problems in arithmetic. They both succumb to the mechanical applications of the right algorithms. My view of inductive inference is less triumphant. Induction is a much muddier activity that often defies the sort of precise characterization given by a neat mathematical formula. It works differently. Inductive inferences are not warranted, I maintain, by conformity with a universal schema, such as those provided by the probability calculus. They are warranted by facts. That is the core claim of the "material theory of induction." In 1903, after much labor, Marie Curie had isolated mere specks of radium chloride crystals. She saw that they belonged to the same crystallographic family as barium chloride. Without hesitation, she inferred that all radium chloride crystals belong there too. What justified the inference? She doesn't say, but it is not hard to know. It is warranted by a fact. A long tradition of work in the 19th century had concluded that, generally, crystalline substances fall into characteristic families according to their chemical constitution. If you have identified the family of one sample--allowing for the inductive risk hiding in the "generally"--you have identified them for all. Now the second trouble: if there is a classic, insoluble problem in philosophy, it is _the_ problem of induction. That is the problem of finding a justification of inductive inference, free of circularity or an infinite regress. If you justify inductive inference by inductive inference, you are trapped in a vicious circle. If you break up the inductive inferences into distinct rules and use one to justify another, you trigger an infinite regress. This problem is philosophy's problem of squaring the circle, the perpetual motion machine, the ether-theory-that-refutes-Einstein. Any proposal to realize or solve them, we know from the outset, is doomed. The only reason one might sit and listen to them is politeness to a speaker. Even then a seat near the door is the best choice. My talk combines both troubles. I argue that adopting the material theory of induction so changes things that the original problem of induction can no longer be set up. The justification of any specific inductive inference lies in other facts. There's no circularity. The fanciful justification of inductive inference rules by others; and others; and still others; is replaced by a prosaic exploration of how this fact in science justifies that; and so on. It is that simple. Or so I say. But more needs to be said. The standard objection to this dissolution is one I've heard many times. Isn't there another fatal regress in this litany of facts justifying facts justifying facts? We trace the chains back to singular observational facts. Using them alone, we have no general fact to warrant the first inductive generalization. The inductive enterprise cannot get started. This objection fails, I urge, because it presumes a narrowly hierarchical picture of inductive support. Each proposition is supposed only to derive support from those less general. They are like stones in a tower that must be supported by those closer to the ground. My comeback exploits this tower metaphor. That is not how things are with relations of inductive support. They are more like those in an arch in which each stone is supported by those lower down and those above it as well. In science, relations of inductive support cross over in a bewildering tangle. This idea gives me the chance to display structures in architecture, I've collected photos of some impressive Roman aqueducts. But my favorite is this vaulted ceiling. (Can anyone say where it is?!) There's more to say. If you haven't had enough, there's a paper "A Material Dissolution of the Problem of Induction." We were now moving to question time. People seemed happy enough, I thought as Carrie Figdor, the chair, fielded the first question for me. Still I knew that this is when I would learn whether my efforts to connect and convince had worked; or if double trouble is just two troubles too many. There were questions whose purpose was, genuinely, to understand better what I was up to. For them I was grateful. There were fewer of them. Then there is another sort of question. I'd quite expected it. Moments before the talk, I'd sketched the familiar protocol to Greg Gandenberger. "The paper is on X. I have a view on X. So I listen up to the moment when the speaker's view diverges from it. Then the exercise becomes how best to ask why the speaker doesn't proceed at that juncture just as I do." The question is not quite what it seems. It is really, "Why don't you see X just the way I do?" When such a question comes, it can be quite hard to know that this is its basis. I had at least one of these. "Why does everything need support?" was the upshot of question. I suspected but didn't know until later in the day what was behind the question. Then I received a cheerful follow up email asking what I thought was wrong with the questioner's resolution of the problem of induction. It just happened to be based directly on the notion that not everything needs support. Then there are questions whose clear intent is to challenge. When there is a genuine disagreement, they should be asked. This is the venue in which such differences are aired and explored. This is how it should be. Allan Franklin, our resident Bayesian for the year, attended the talk, but missed questions since he had a plane to catch--truly. He commissioned John Earman to ask the Bayesian question for him; and John asked it thoroughly and with polish. Why isn't the solution of the problem just an application of probabilistic mathematics? Conditionalize on a history of black ravens and, if the priors and likelihoods are right, you have all ravens are black, with high probability. It's just a theorem of mathematics. (My answer: there are circumstances in which the background material facts do not warrant a probabilistic logic, so there's no universally applicable probabilistic, inductive logic and hence it cannot give a solution to the problem of induction.) Finally there are questions that dispute, even though the questioner has no real disagreement. Rather they seem to feel an obligation to make the speaker work, to test his mettle, to put him through his paces, as though he was a racehorse who had taken too lazy a trot round the course and needed to be made to work and sweat. They find some part of the talk where they think things are soft and they press to see how far it yields.
Why, I often wonder, don't we replace that protocol with another? If you've found a soft spot and have no competing view to defend, why not put your efforts into figuring out how to firm up the speaker's view? You will still get to ask your question. You can display your erudition, if that is your goal, and the speaker will appreciate the effort.
"How do you think it went? Was it OK?" I knew immediately from the long pause and look of alarm that it was a bad question to ask. She struggled to find some easy way to let me down. "What do you mean?... You didn't convince anyone..." "Oh no," I said, "I _never_ expected that..." Then she proceeded to reassure me that this talk had a much worthier target than the last one I gave. Faint praise, but I'll take it. Of course I lied. I had hoped to convince someone. It isn't an unreachable goal. But success depends on more than my content and my limited skill at presentation. Of that I was now sure. A talk is an interaction and the audience plays their part. I'd given the exact same talk a few weeks before at a conference in Madrid on inferentialism in philosophy and philosophy of science. There were the usual challenges then in question time, following something like the protocols above. But there was something else. There was a strong presence with real philosophical gravitas. It was willing to tell me that I was right, and quite obviously right; and eager to suggest ways that I could strengthen the argument.
John D. Norton
|